Chapter 1: The Algebraic Puzzle

Have you ever pondered how much income one truly needs for a fulfilling life? Of course, this varies greatly based on personal definitions of "fulfilling." Recently, this question has been on my mind, but let's set that aside for now. For the moment, let's focus on manipulating the expression presented above and identifying the greatest power of 2 that can be factored out.

I encourage you to pause here, grab a pen and paper, and tackle this challenge yourself. Once you're ready, continue reading for the solution!

To solve this puzzle, a little intuition goes a long way. Notice that (1002/2 = 501), which allows us to rewrite the expression as follows:

We can extract the common factor (2^1).

The next step involves applying the difference of squares formula: (a^2 - b^2 = (a + b)(a - b)). To achieve this, we need to recognize that (5^{1/2} = (5^{1/4})^2).

In this scenario, (a = 5^{1/4}) and (b = 1). Here lies the trickiest part of the puzzle. We aim to further simplify the terms ((5^{1/4} + 1)) and ((5^{1/4} - 1)) to uncover any hidden powers of 2.

Since both ((5^{1/4} + 1)) and ((5^{1/4} - 1)) are even, they must contain some powers of 2. Let's first analyze ((5^{1/4} + 1)):

We rewrite (5^{1/4}) using two alternating sequences of powers of 5. This allows us to factor out a 5 in the first series, which can then be expressed as ((5 + 1)) multiplied by an odd number of terms.

We will use a similar approach for ((5^{1/4} - 1)):

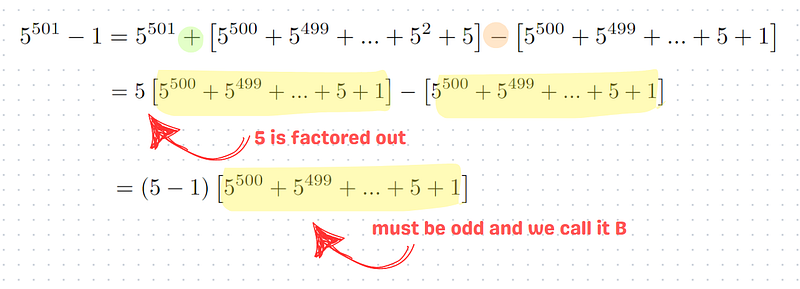
This manipulation is arguably more straightforward. We denote the two odd sums with odd terms as (A) and (B).

Now, let’s compile everything into one coherent expression:

At this point, we can disregard (A) and (B) because they are odd. The product of two odd numbers remains odd. We know that ((5 + 1)(5 - 1) = 24 = 2^3(3)), thus yielding our final answer.

P.S. Crafting this puzzle took considerable time and effort, especially in explaining the terms ((5^{1/4} + 1)) and ((5^{1/4} - 1)).

How fascinating! What was your thought process during this challenge? Please share your insights in the comments—I’m eager to hear from you!

Chapter 2: Video Insights

In this chapter, we explore two insightful videos that delve deeper into the concept of powers of 2.

The first video, titled "What is the largest power of 2 that divides this?" provides an engaging exploration of the topic. It guides viewers through the thought process behind identifying the largest power of 2 in various expressions.

The second video, "Index Greatest Power [Numbers]," continues the discussion, presenting different perspectives and techniques for approaching similar problems.